题目描述:
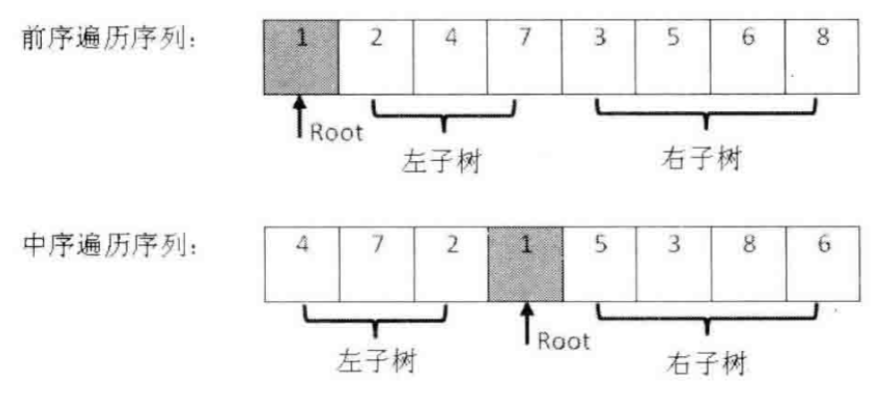
输入某二叉树的前序遍历和中序遍历的结果,请重建出该二叉树。假设输入的前序遍历和中序遍历的结果中都不含重复的数字。例如输入前序遍历序列{1,2,4,7,3,5,6,8}和中序遍历序列{4,7,2,1,5,3,8,6},则重建二叉树并返回根结点。
解题思路:
通常树有如下几种遍历方式:
- 前序遍历:先访问根结点,再访问左子结点,最后访问右子结点。
- 中序遍历:先访问左子结点,再访问根结点,最后访问右子结点。
- 后序遍历:先访问左子结点,再访问右子结点,最后访问根结点。
本题为前序遍历和中序遍历,最少需要两种遍历方式,才能重建二叉树。
本题是根据前序和中序遍历序列重建二叉树,我们可以通过一个具体的实例来发现规律,不难发现:前序遍历序列的第一个数字就是树的根结点。在中序遍历序列中,可以扫描找到根结点的值,则左子树的结点都位于根结点的左边,右子树的结点都位于根结点的右边。
这样,我们就通过这两个序列找到了树的根结点、左子树结点和右子树结点,接下来左右子树的构建可以进一步通过递归来实现。
举例:
代码实现(c++)
/**
* Definition for binary tree
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode(int x) : val(x), left(NULL), right(NULL) {}
* };
*/
class Solution {
public:
TreeNode* reConstructBinaryTree(vector<int> pre,vector<int> vin) {
if(pre.size() == 0){ //如果为空,返回NULL
return NULL;
}
//依次是前序遍历左子树,前序遍历右子树,中序遍历左子树,中序遍历右子树
vector<int> left_pre, right_pre, left_vin, right_vin;
//前序遍历第一个节点一定为根节点
TreeNode* head = new TreeNode(pre[0]);
//找到中序遍历的根节点
int root = 0;
//遍历找到中序遍历根节点索引值
for(int i = 0; i < pre.size(); i++){
if(pre[0] == vin[i]){
root = i;
break;
}
}
//利用中序遍历的根节点,对二叉树节点进行归并
for(int i = 0; i < root; i++){
left_vin.push_back(vin[i]);
left_pre.push_back(pre[i + 1]); //前序遍历第一个为根节点
}
for(int i = root + 1; i < pre.size(); i++){
right_vin.push_back(vin[i]);
right_pre.push_back(pre[i]);
}
//递归,再对其进行上述所有步骤,即再区分子树的左、右子子数,直到叶节点
head->left = reConstructBinaryTree(left_pre, left_vin);
head->right = reConstructBinaryTree(right_pre, right_vin);
return head;
}
};
代码实现(java)
public TreeNode reConstructBinaryTree(int [] pre,int [] in) {
/*根据前序遍历和中序遍历确定一棵二叉树*/
//递归实现
if(pre==null||in==null||pre.length==0)
return null;
return reConstructBinaryTree(pre,in,0,pre.length-1,0,in.length-1);
}
public TreeNode reConstructBinaryTree(int [] pre,int [] in,int pre_begin,
int pre_end,int in_begin,int in_end)
{
////前序序列:从pre_begin到pre_end, 中序序列:从in_begin到in_end
//递归结束条件
if(pre_begin>pre_end || in_begin>in_end)
return null;
int rootValue=pre[pre_begin];
TreeNode root=new TreeNode(rootValue); //第一个节点就是根节点
if(pre_begin==pre_end || in_begin==in_end)
return root;
//在中序序列中,找到root,前面的就是左子树,右边的就是右子树
int rootIn=in_begin; //root在中序序列中的位置
while(rootIn<=in_end && in[rootIn]!=rootValue)
rootIn++;
int left_count=rootIn-in_begin; //左子树节点个数
root.left=reConstructBinaryTree(pre,in,pre_begin+1,pre_begin+left_count,
in_begin,rootIn-1);
root.right=reConstructBinaryTree(pre,in,pre_begin+left_count+1,
pre_end,rootIn+1,in_end);
return root;
}
代码实现(python2.7)
# -*- coding:utf-8 -*-
# class TreeNode:
# def __init__(self, x):
# self.val = x
# self.left = None
# self.right = None
class Solution:
# 返回构造的TreeNode根节点
def reConstructBinaryTree(self, pre, tin):
# write code here
if len(pre) == 0:
return None
elif len(pre) == 1:
return TreeNode(pre[0])
else:
root = TreeNode(pre[0])
pos = tin.index(pre[0])
root.left = self.reConstructBinaryTree(pre[1:pos+1], tin[:pos])
root.right = self.reConstructBinaryTree(pre[pos+1:], tin[pos+1:])
return root
 菜鸟笔记
菜鸟笔记